Balloon Balance Puzzles

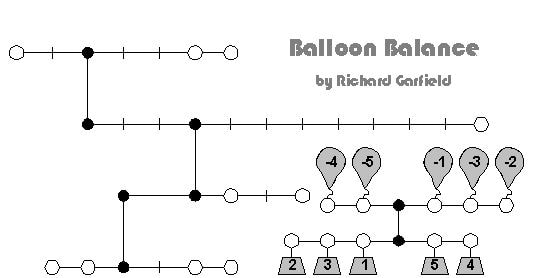
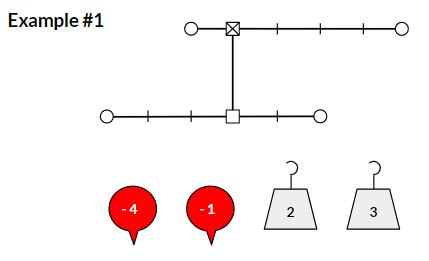
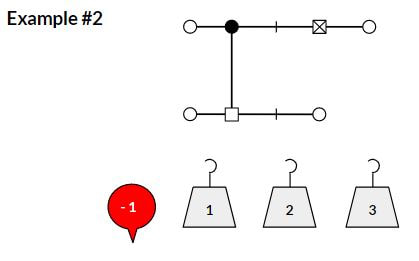
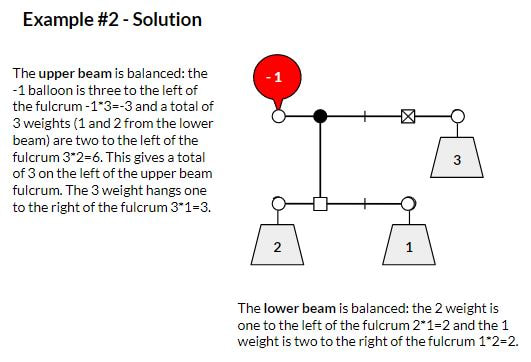
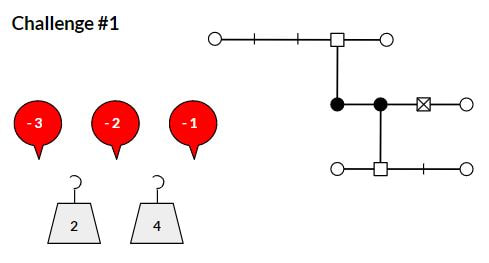
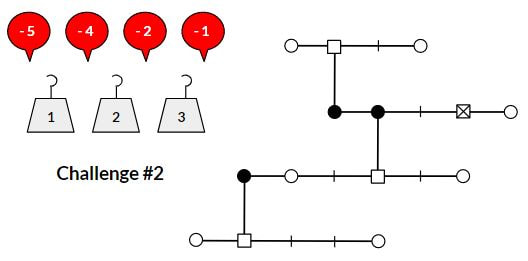
I recently stumbled across Richard Garfield's Balloon Balance puzzle from the 9th World Puzzle Championship in October, 2000. The puzzle is similar traditional balance puzzles such as SolveMe Mobiles but with a couple of twists. The first twist is the use of balloons to include negative numbers. The second twist is the inclusion of torque... the farther from the balance point (i.e. fulcrum), the more force is applied.

I thought that a simplified version of this puzzle might work well for students  who are practicing integer multiplication. First however I had to figure out the puzzle. I have to admit that this puzzle took me quite a while to solve and make sense of. I almost gave up a couple of times but I eventually figured it out. Solving this puzzle reminded me of how it feels to be a learner and to empathize with students struggling to understand a new concept. If you'd like to see the solution to the puzzle above, click this link. 

To use this with students, I simplified the puzzle a bit and then made some examples and easier challenges to start with. I modeled these from a version of this puzzle created by Joseph DeVincentis for the Boston Area Puzzle Hunt League (BAPHL) #5.

Directions: On each set of balances, place the given balloons and weights in the open circles. Each beam should be balanced around its fulcrum (the small square) by the torque rule: sum of weight times distance from fulcrum for all weights on the left should equal that on the right. The beams themselves are weightless. The fulcrum with an x is the fixed anchor point for the system.

The first example shows students how the basic puzzle works. The second example below add additional complexity to the system while retaining only 4 weights/balloons to place.

I created two challenge puzzles for students to work on once they were confident with the examples.

I haven't tried this out with students yet so I'm not sure what to expect. Feel free to check out my Google Slides with the examples, challenges and solutions. I'd appreciate feedback if you have suggestions to improve this.

Nova Scotia Mathematics Curriculum Outcomes 
Grade 8 N07 - Students will be expected to demonstrate an understanding of multiplication and division of integers, concretely, pictorially, and symbolically.

EL