 https://www.ted.com/ https://www.ted.com/ I am trying to be purposeful and organized in taking the "Calls to Action" from the recent NCTM conference in San Francisco. I am going to play with "Deleting the Texbook" over my next few blog posts (watch Meyer's most recent NCTM presentation here for elaboration). Since I attended my first conference about 7 years ago, I have been heavily influenced by the work of Dan Meyer. His Ted Talk "Math Class Needs a Makeover" really changed the way I thought about teaching math.  I want to share something I did with first as a math coach with a class of grade 3 students and then as a presentation for teachers at the Nova Scotia MTA conference a few year back. I feel like I have been taking this particular "Call to Action" for many years now; I am excited about the evolution of this thinking and I wanted to show how I started with this work. The students in this class were a few weeks out from writing a provincial math assessment. The classroom teacher wanted to do some questions from the practice booklet. This was the original question:  Instead of posing the question as is, I took out as much information as possible while keeping the math I wanted the students to learn in mind. The students needed to get curious before I hit them with the math. All staff at schools need to wear an ID badge and laynard (wait for it...it will make sense in a moment). Lots of people keep keys, change, notes, etc in the plastic badge holder. Instead of asking the above question, I announced to the class that I had some change in my ID holder (I put the correct amount in and shook it for dramatic affect!). Their job was to figure out how much money I had. They were a little confused at first, so I prompted them; I told them they could ask me questions about the coins. I gave them a few minutes to think, then to confer with a partner before I took questions from the class. I recorded and answered their questions. Here are the questions they came up with along with my answers in brackets:
Each time I took a question, I asked students "Why do you want to know that?" or "How will that information help you solve the problem?" Once they had all the information they felt they needed, they set off to solve the problem. They basically constructed the original question on their own. And because they had a part in building it, they really understood the context of the problem and had ideas of how they might solve it. When they were confident that they had the solution, I let them empty my ID badge and count the coins. That is much more satisfying than just giving the correct answer. Students got to prove that they were right, rather than the teacher being in charge of all the answers. I often hear from teachers that their students don't understand word problems and that it's not the math, it's reading comprehension. I see this strategy of building a question with the class as a way to help them get inside the problem before they start. Another reason I like this strategy so much is that I was able to create it by modifying a resource (practice booklet) that I already had. We can get bogged down trying to find appropriate resources for students at appropriate levels. By using a resource I already had, taking out parts, and layering them back in as needed I was able to differentiate on the fly. No tiered lesson plan, no separate handouts. It was one problem that we worked together as a class to first build, then solve. The Most Interesting Question I Almost Overlooked"What kind of coffee do you drink?
I didn't understand where the student was going with the question but I asked the usual questions of him: "How will that help you solve the problem?" and "Why do you want to know?" The student thought I probably kept my coffee money in my ID badge. If he knew the coffee I drank, he would have the problem solved.** Based on his question, we created a series of extension questions to keep the problem solving going. **All Canadians know the price of a medium double double. :P KZ |
Categories
All
|
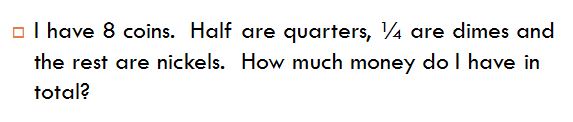
 RSS Feed
RSS Feed